+ - 0:00:00
Notes for current slide
Notes for next slide

2.6: Long Run Industry Equilibrium

ECON 306 · Microeconomic Analysis · Spring 2020

Ryan Safner
Assistant Professor of Economics
safner@hood.edu
ryansafner/microS20
microS20.classes.ryansafner.com

Firm's Long Run Supply Decisions

Firm Decisions in the Long Run I

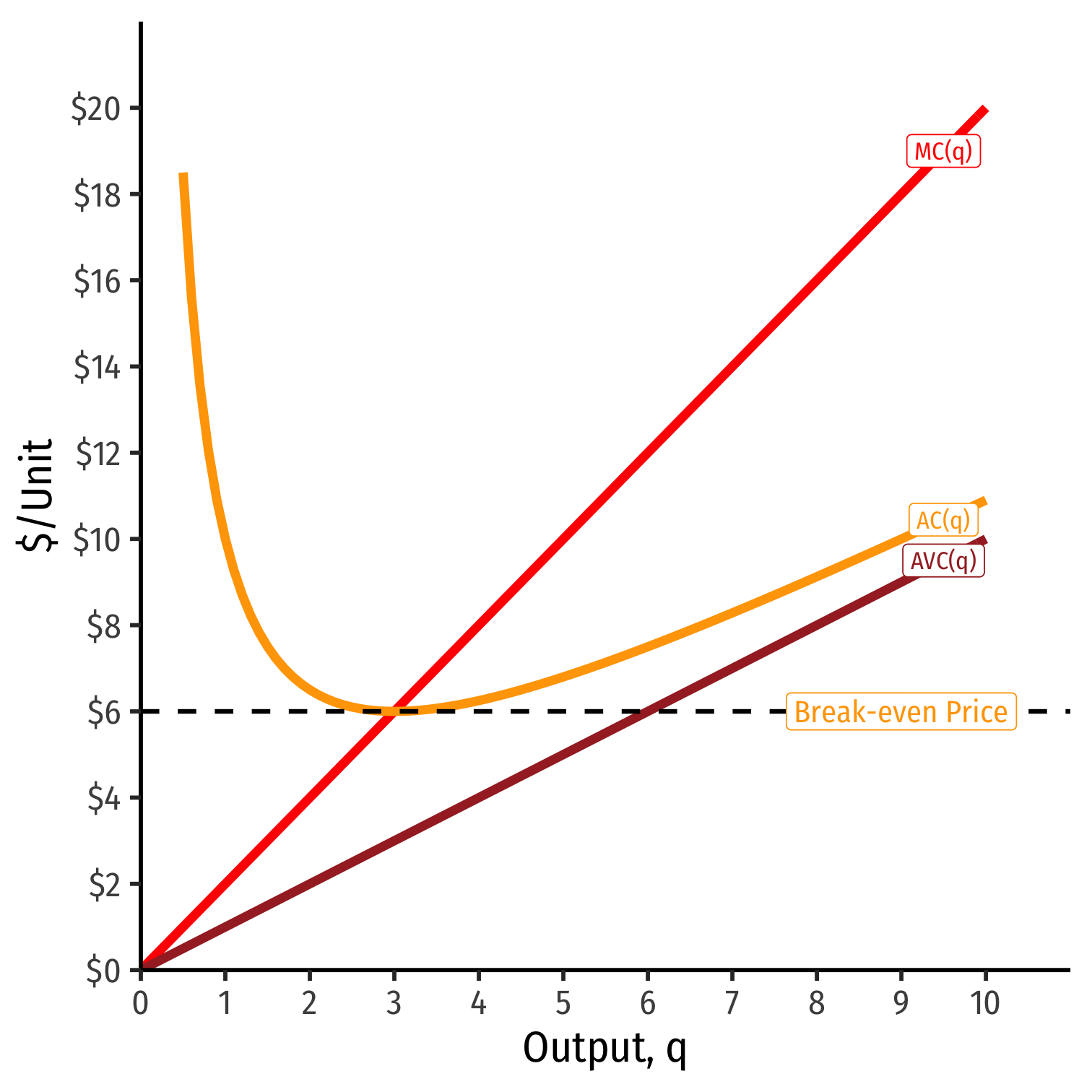
  • AC(q)min at a market price of $6

  • At $6, the firm earns "normal economic profits"

  • At any market price below $6.00, firm earns losses

    • Short Run: firm shuts down if p<AVC(q)

  • At any market price above $6.00, firm earns "supernormal profits"

Firm Supply Decisions in the Short Run vs. Long Run

  • Short run: firms that shut down (q∗=0) stuck in market, incur fixed costs π=−f

Firm Supply Decisions in the Short Run vs. Long Run

  • Short run: firms that shut down (q∗=0) stuck in market, incur fixed costs π=−f

  • Long run: firms earning losses (π<0) can exit the market and earn π=0

    • No more fixed costs, firms can sell/abandon f at q∗=0

Firm Supply Decisions in the Short Run vs. Long Run

  • Short run: firms that shut down (q∗=0) stuck in market, incur fixed costs π=−f

  • Long run: firms earning losses (π<0) can exit the market and earn π=0

    • No more fixed costs, firms can sell/abandon f at q∗=0

  • Entrepreneurs not currently in market can enter and produce, if entry would earn them π>0

Firm's Long Run Supply: Visualizing

When p<AVC

  • Profits are negative

  • Short run: shut down production

    • Firm loses more π by producing than by not producing

  • Long run: firms in industry exit the industry

    • No new firms will enter this industry

Firm's Long Run Supply: Visualizing

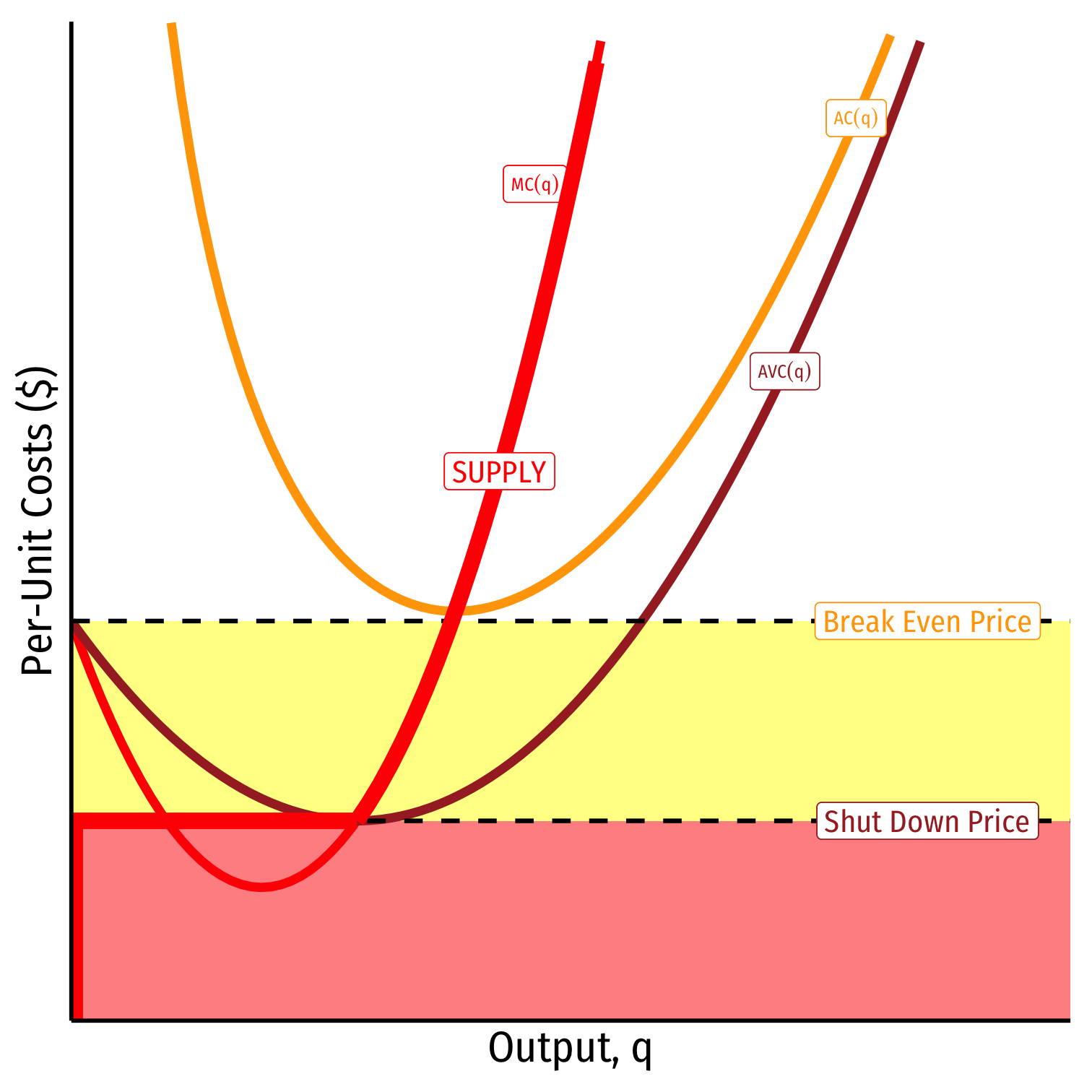
When AVC<p<AC

  • Profits are negative

  • Short run: continue production

    • Firm loses less π by producing than by not producing

  • Long run: firms in industry exit the industry

    • No new firms will enter this industry

Firm's Long Run Supply: Visualizing

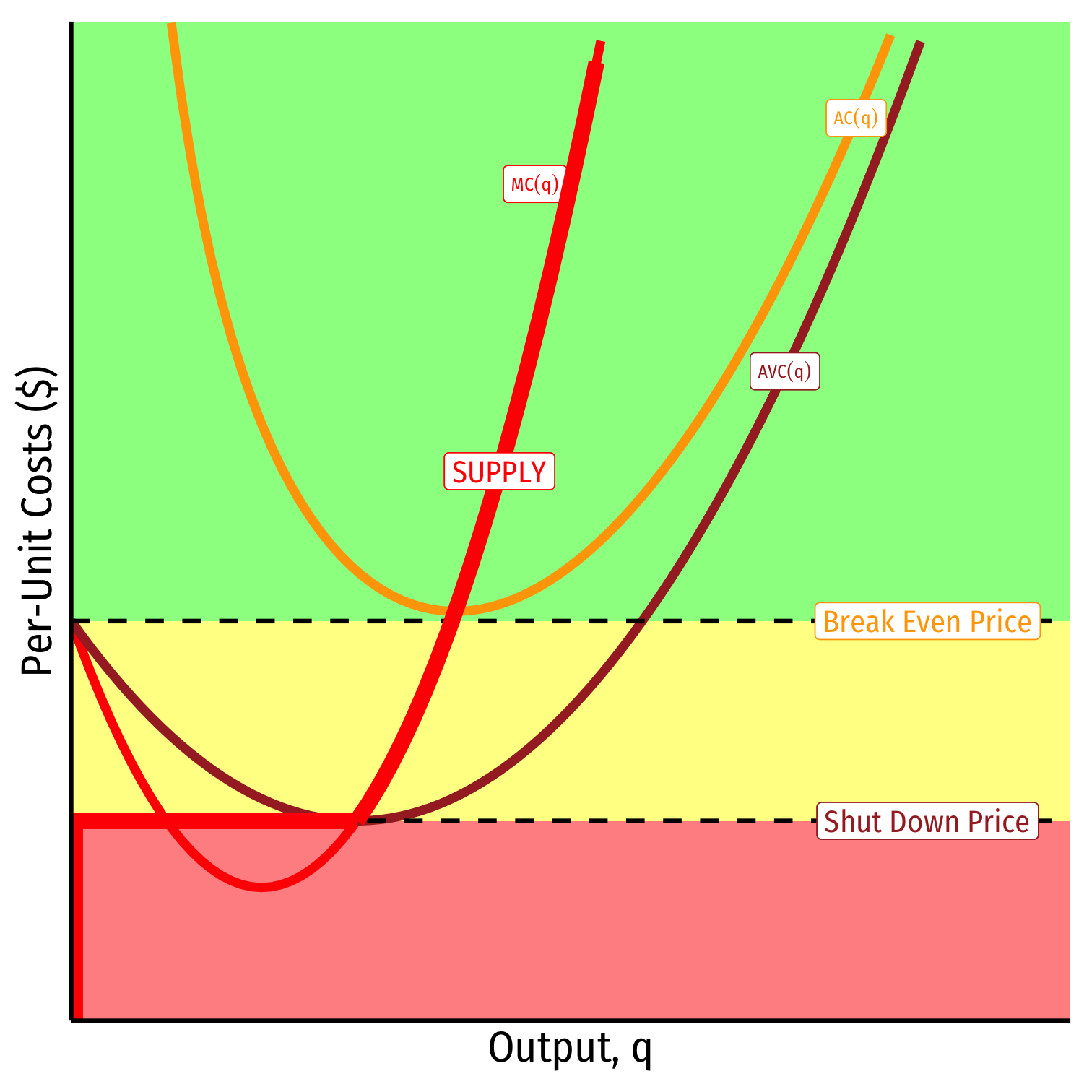
When AC<p

  • Profits are positive

  • Short run: continue production

    • Firm earning profits

  • Long run: firms in industry stay in industry

    • New firms will enter this industry

Production Rules, Updated:

1. Choose q∗ such that MR(q)=MC(q)

2. Profit π=q[p−AC(q)]

3. Shut down in short run if p<AVC(q)

4. Exit in long run if p<AC(q)

Market Entry and Exit

Exit, Entry, and Long Run Industry Equilibrium I

  • Now we must combine optimizing individual firms with market-wide adjustment to equilibrium

  • Since π=[p−AC(q)]q, in the long run, profit-seeking firms will:

Exit, Entry, and Long Run Industry Equilibrium I

  • Now we must combine optimizing individual firms with market-wide adjustment to equilibrium

  • Since π=[p−AC(q)]q, in the long run, profit-seeking firms will:

    • Enter markets where p>AC(q)

Exit, Entry, and Long Run Industry Equilibrium I

  • Now we must combine optimizing individual firms with market-wide adjustment to equilibrium

  • Since π=[p−AC(q)]q, in the long run, profit-seeking firms will:

    • Enter markets where p>AC(q)
    • Exit markets where p<AC(q)

Exit, Entry, and Long Run Industry Equilibrium II

  • Long-run equilibrium: entry and exit ceases when p=AC(q) for all firms, implying normal economic profits of π=0

Exit, Entry, and Long Run Industry Equilibrium II

  • Long-run equilibrium: entry and exit ceases when p=AC(q) for all firms, implying normal economic profits of π=0

  • Zero Economic Profits Theorem: long run economic profits for all firms in a competitive industry are 0

  • Firms must earn an accounting profit to stay in business

Deriving the Industry Supply Curve

The Industry Supply Curve

  • Industry supply curve: horizontal sum of all individual firms' supply curves

    • recall: (MC(q) curve above AVCmin) (shut down price)

  • To keep it simple on the following slides:

    • assume no fixed costs, so AC(q)=AVC(q)
    • then industry supply curve is sum of individual MC(q) curves above AC(q)min

Industry Supply Curves (Identical Firms)









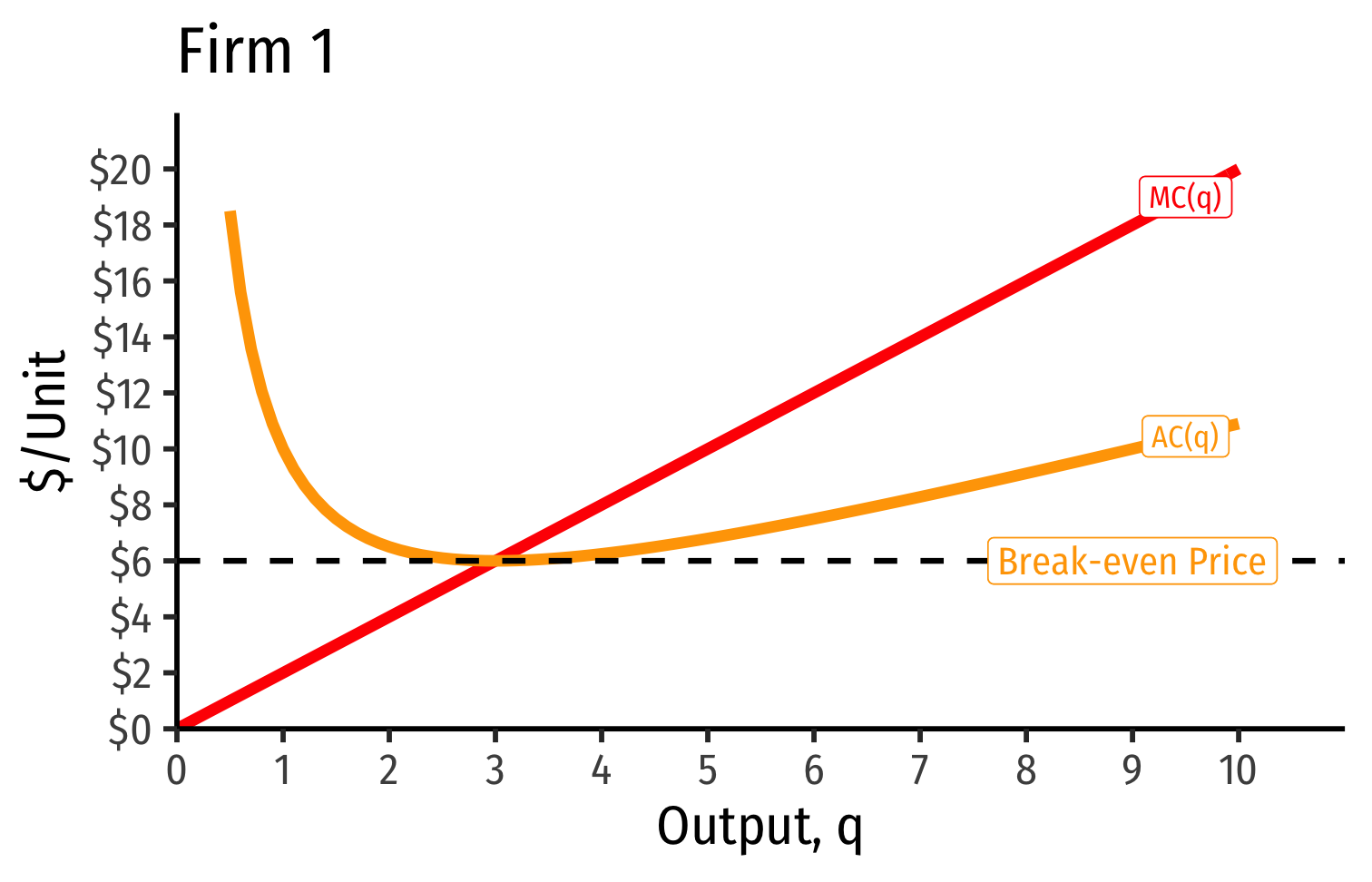
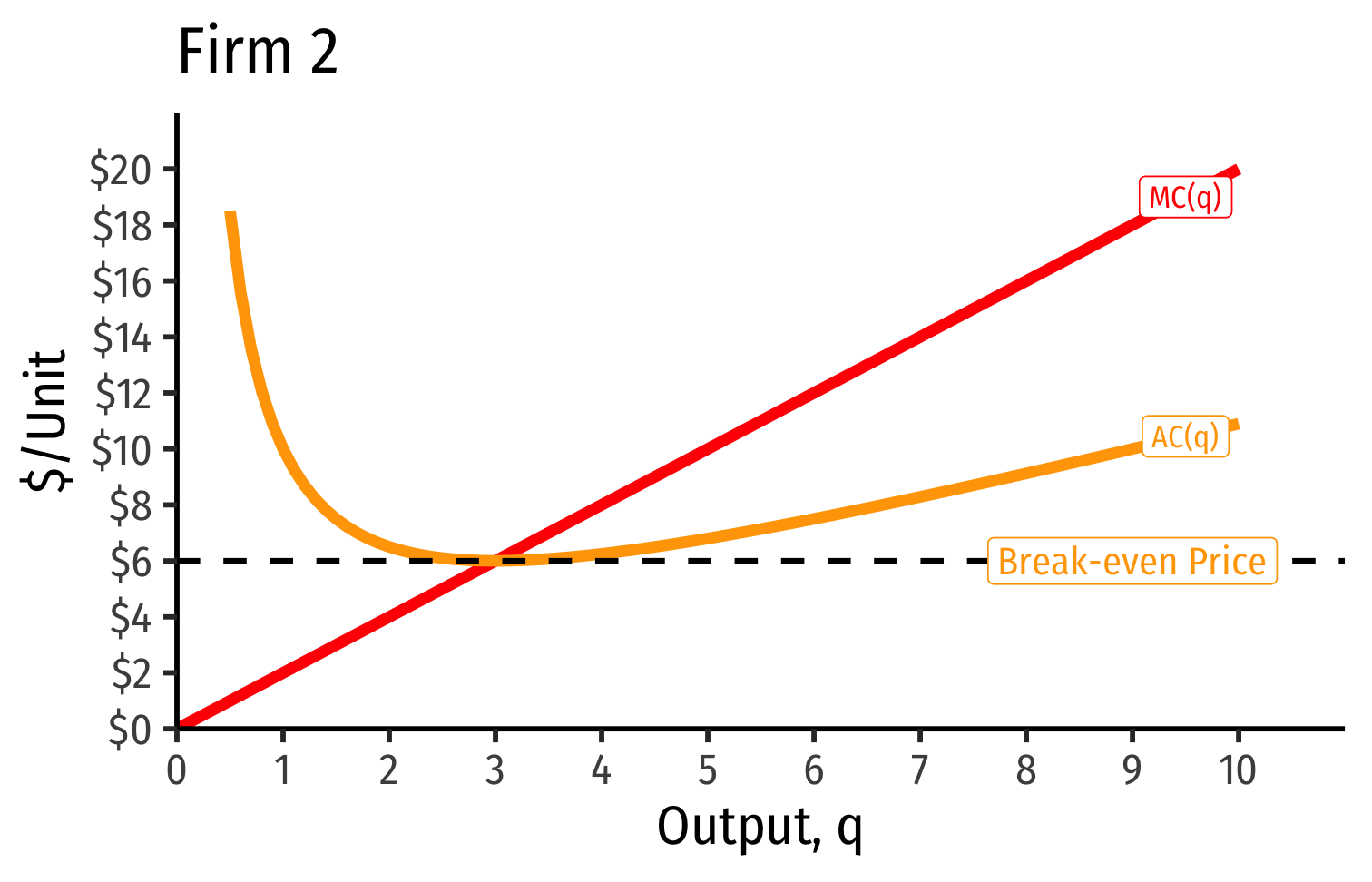
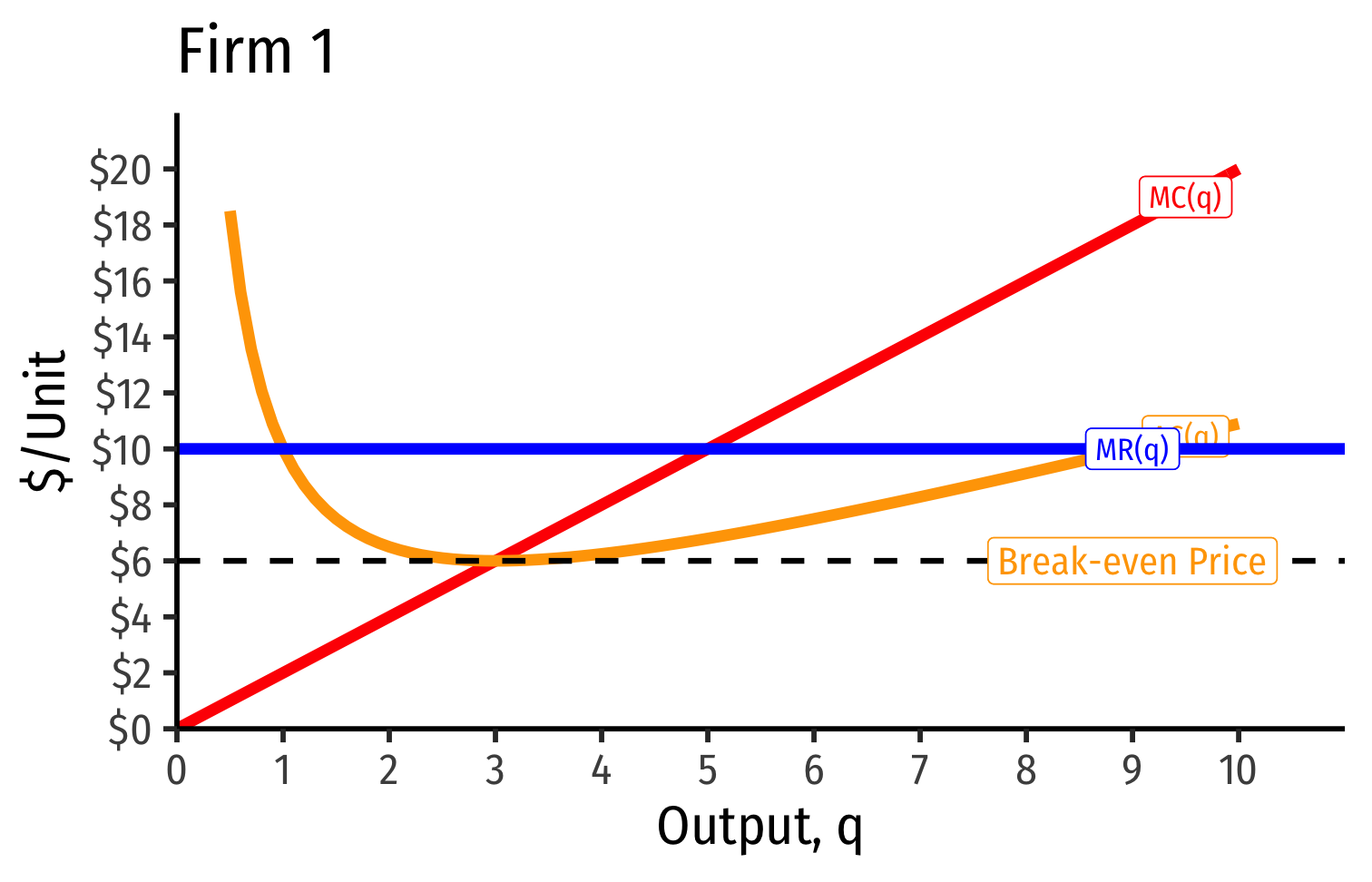
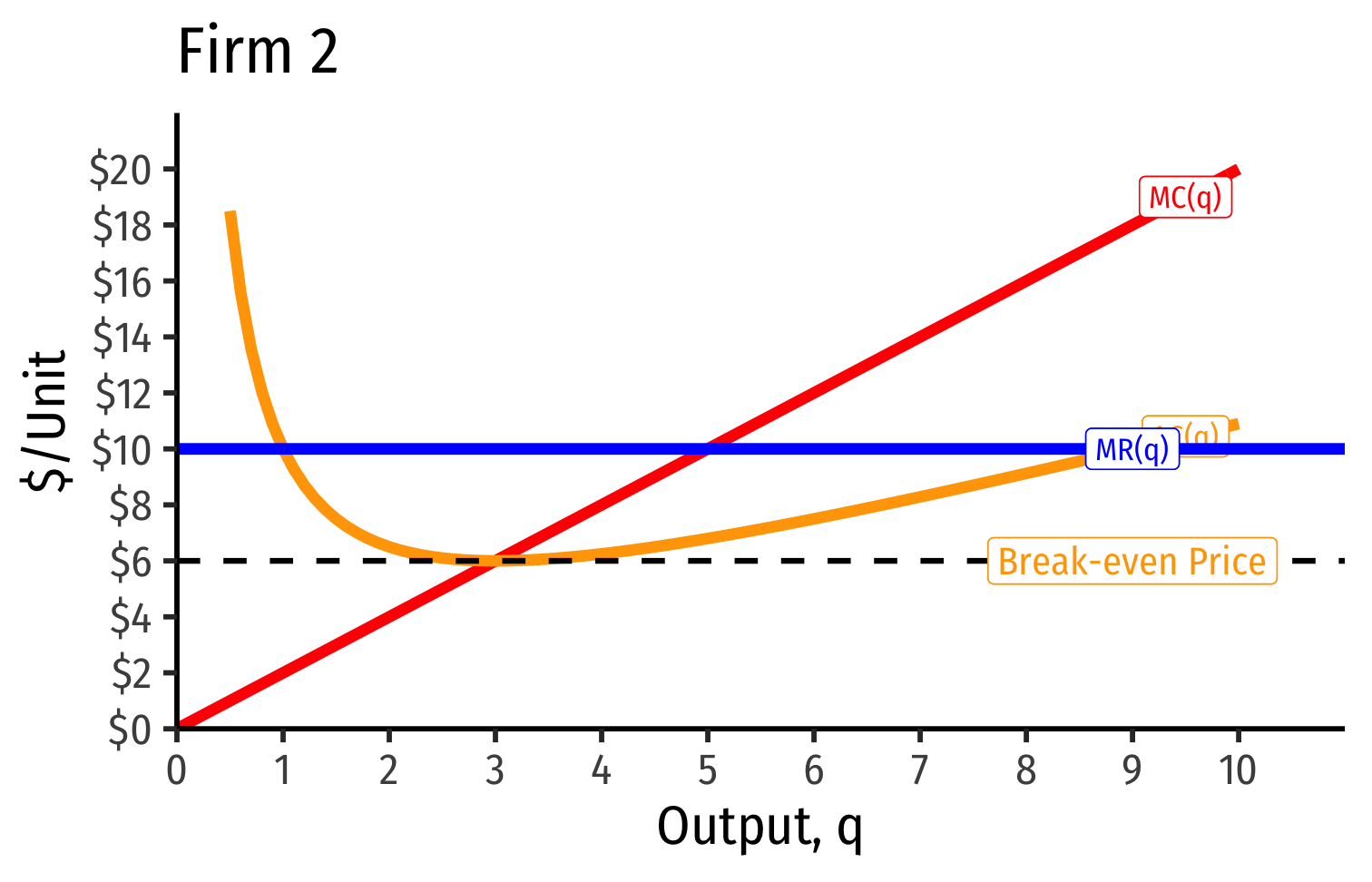
Industry Supply Curves (Identical Firms)









  • Industry supply curve is the horizontal sum of all individual firm's supply curves
    • Which are each firm's marginal cost curve above its breakeven price

Industry Supply Curves (Identical Firms)









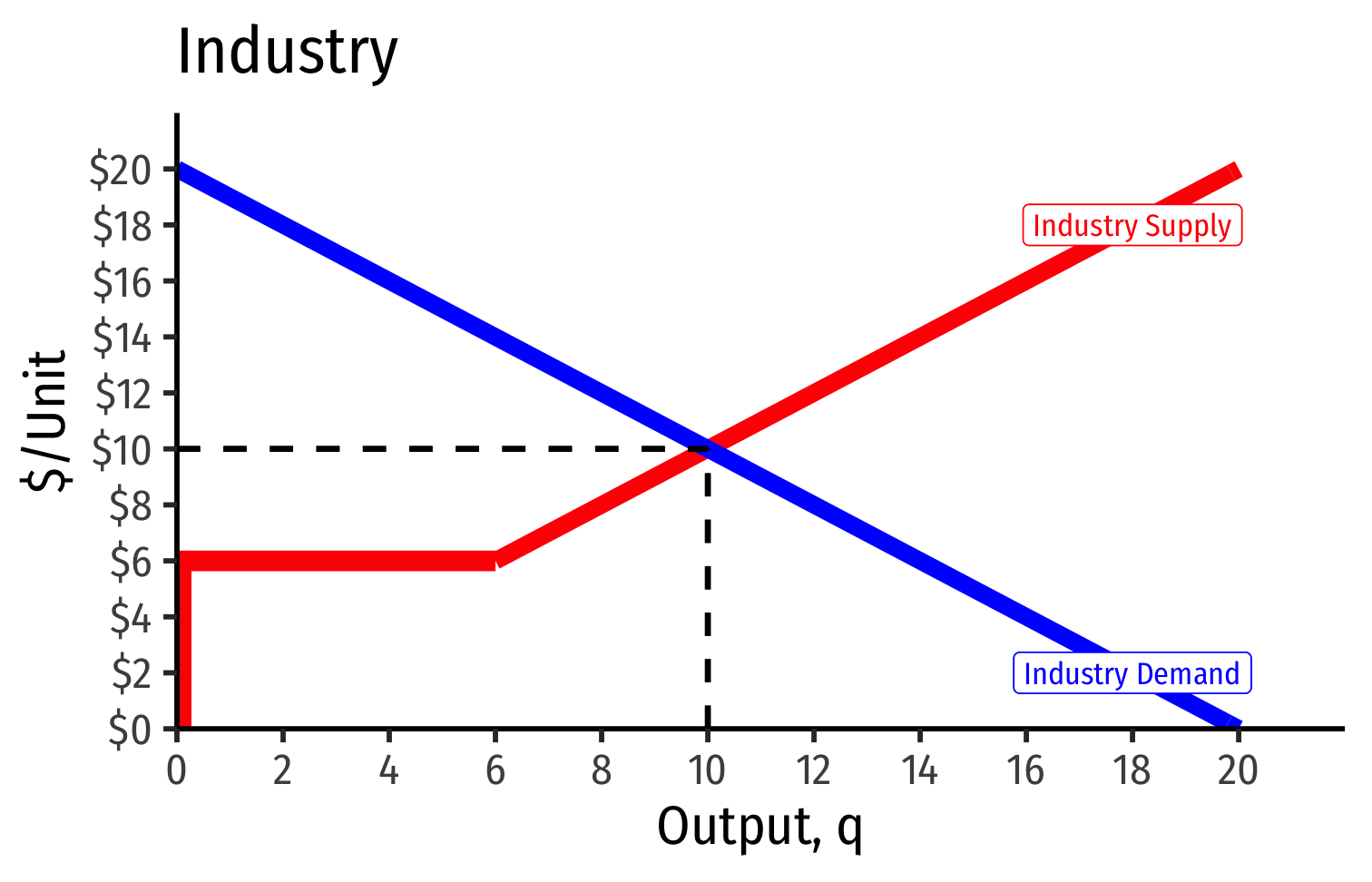
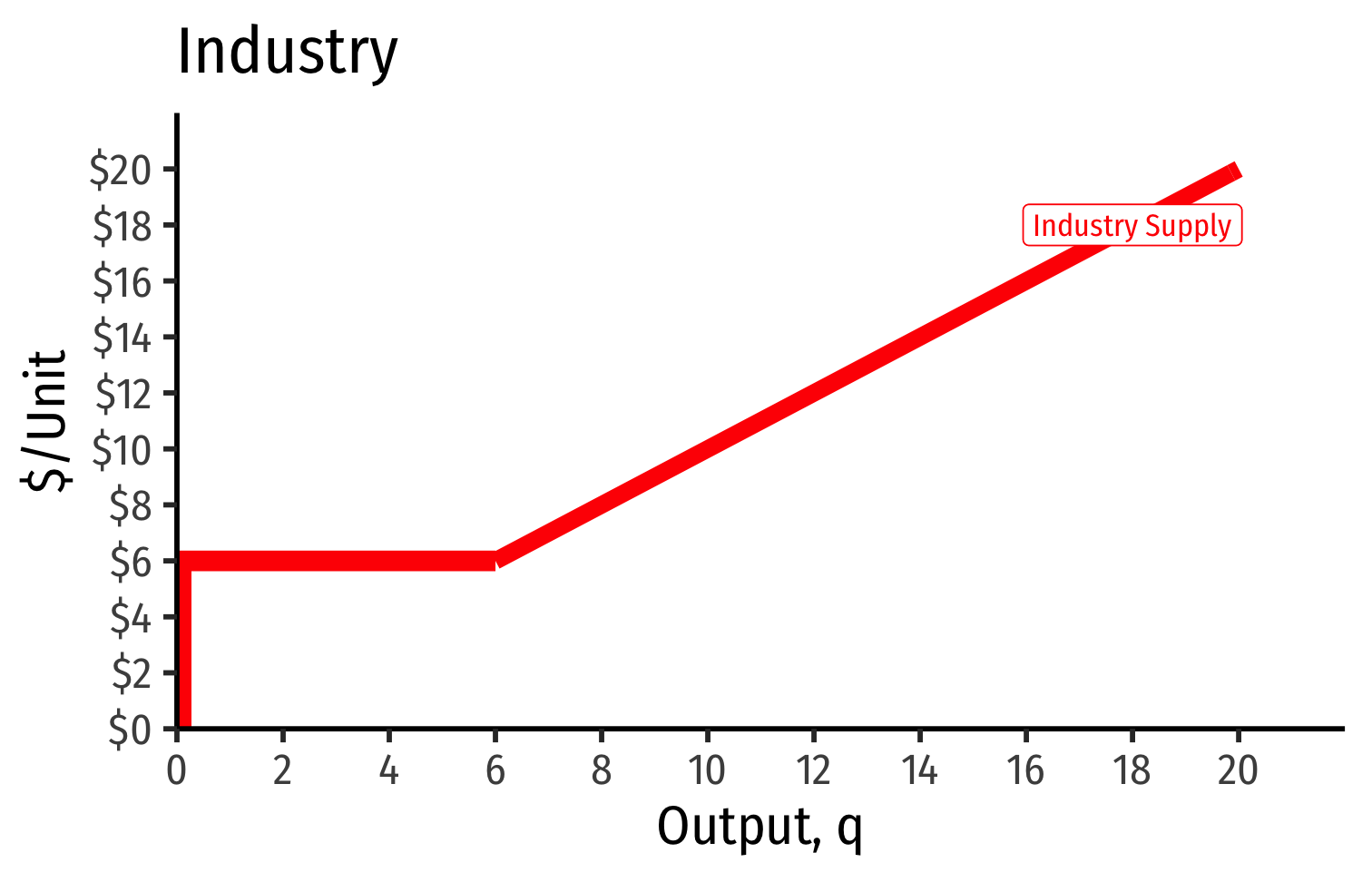
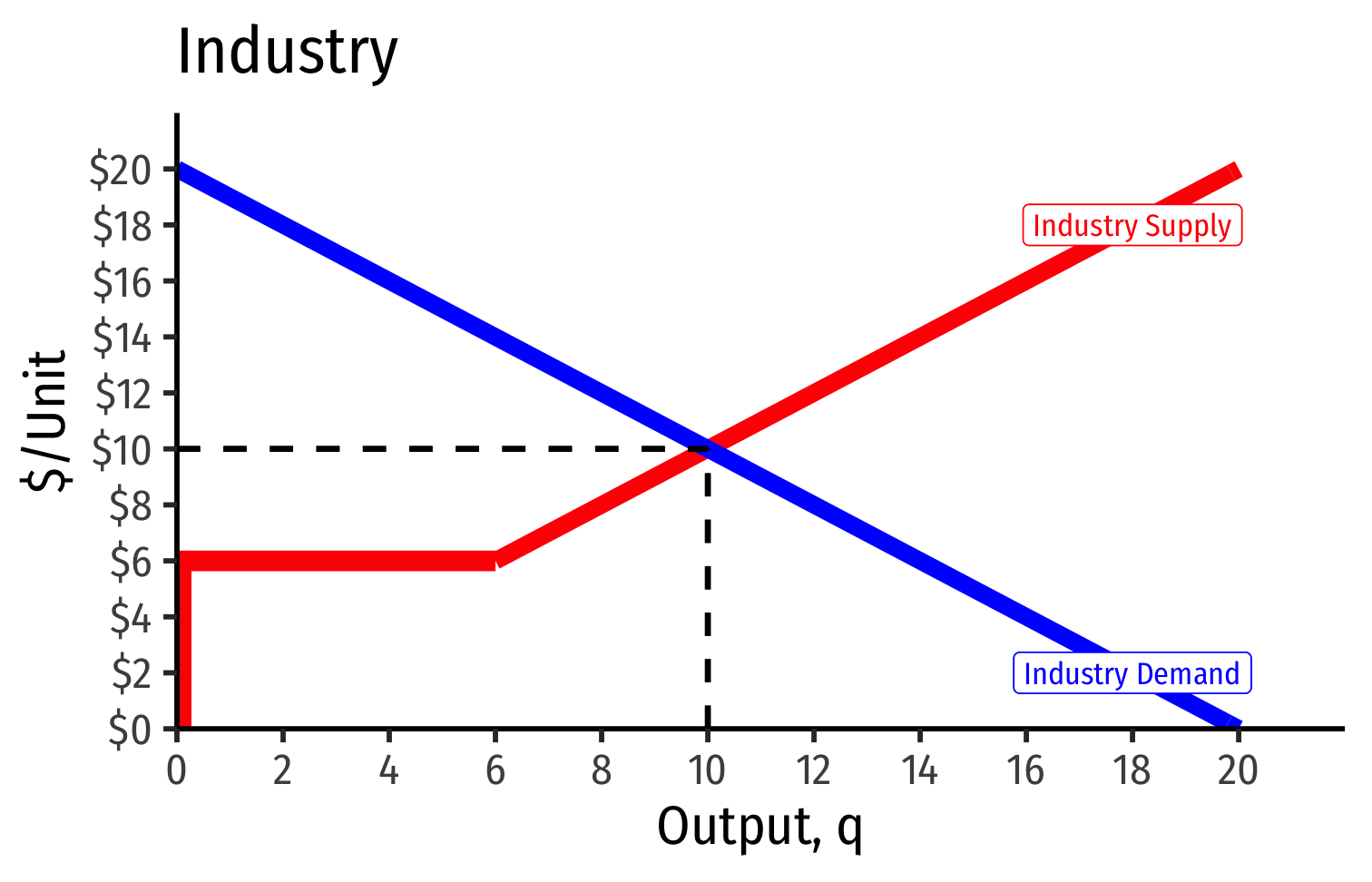
  • Industry demand curve (where equal to supply) sets market price, demand for firms

Industry Supply Curves (Identical Firms)









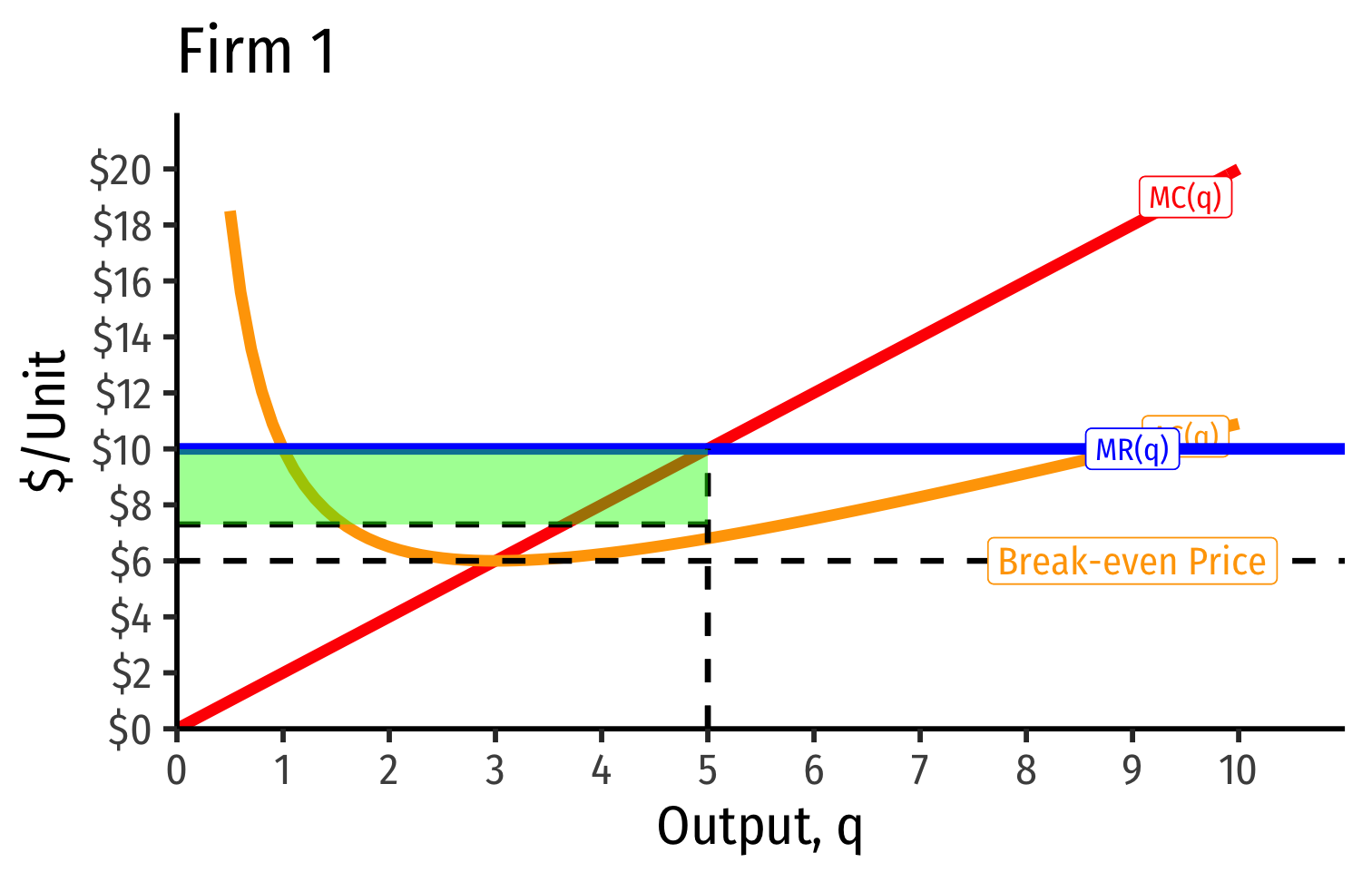
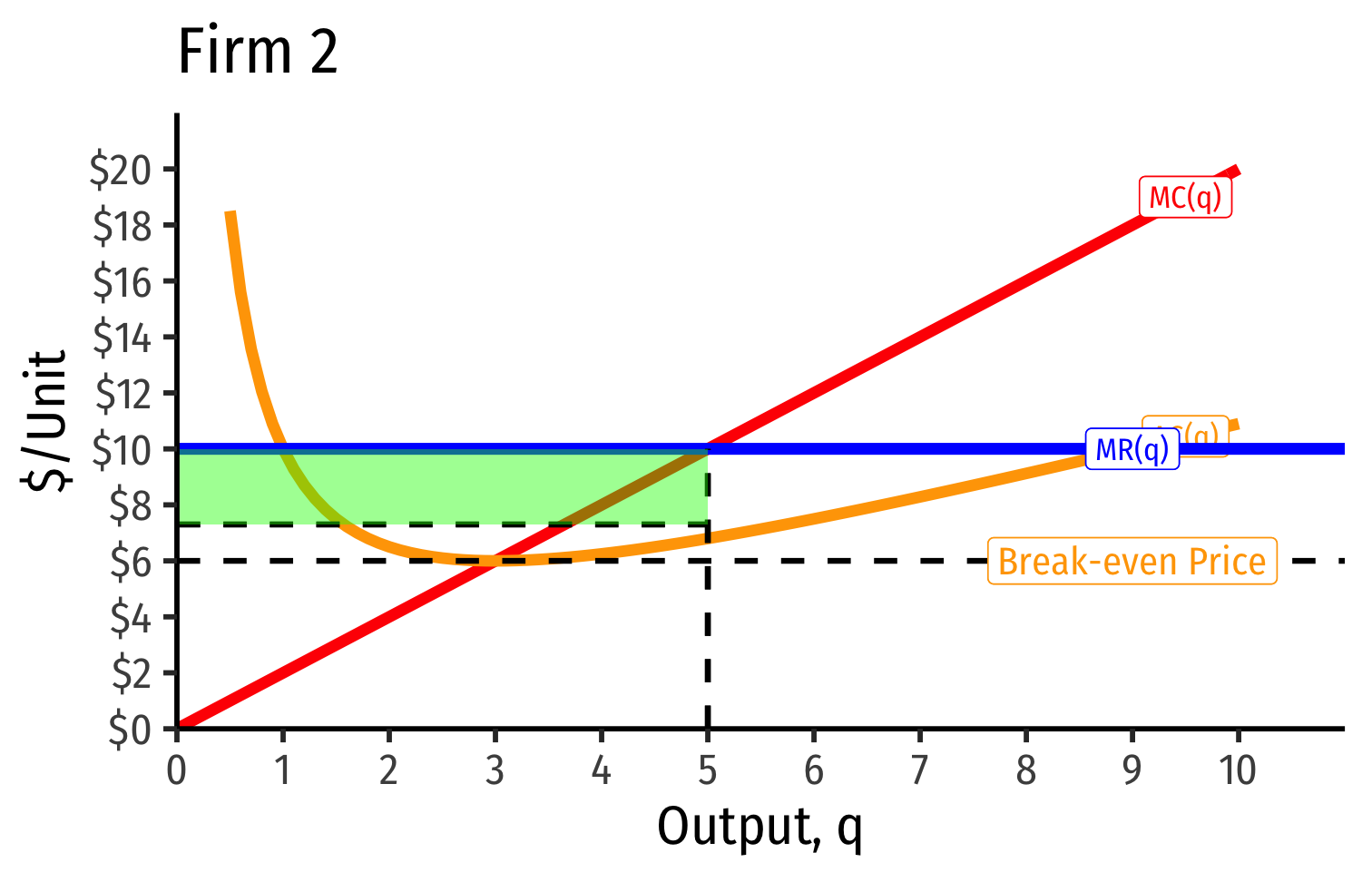
  • Short Run: each firm is earning profits p>AC(q)

  • Long run: induces entry by firm 3, firm 4, ⋯, firm n

Industry Supply Curves (Identical Firms)









  • Short Run: each firm is earning profits p>AC(q)

  • Long run: induces entry by firm 3, firm 4, ⋯, firm n

  • Long run industry equilibrium:

Industry Supply Curves (Identical Firms)









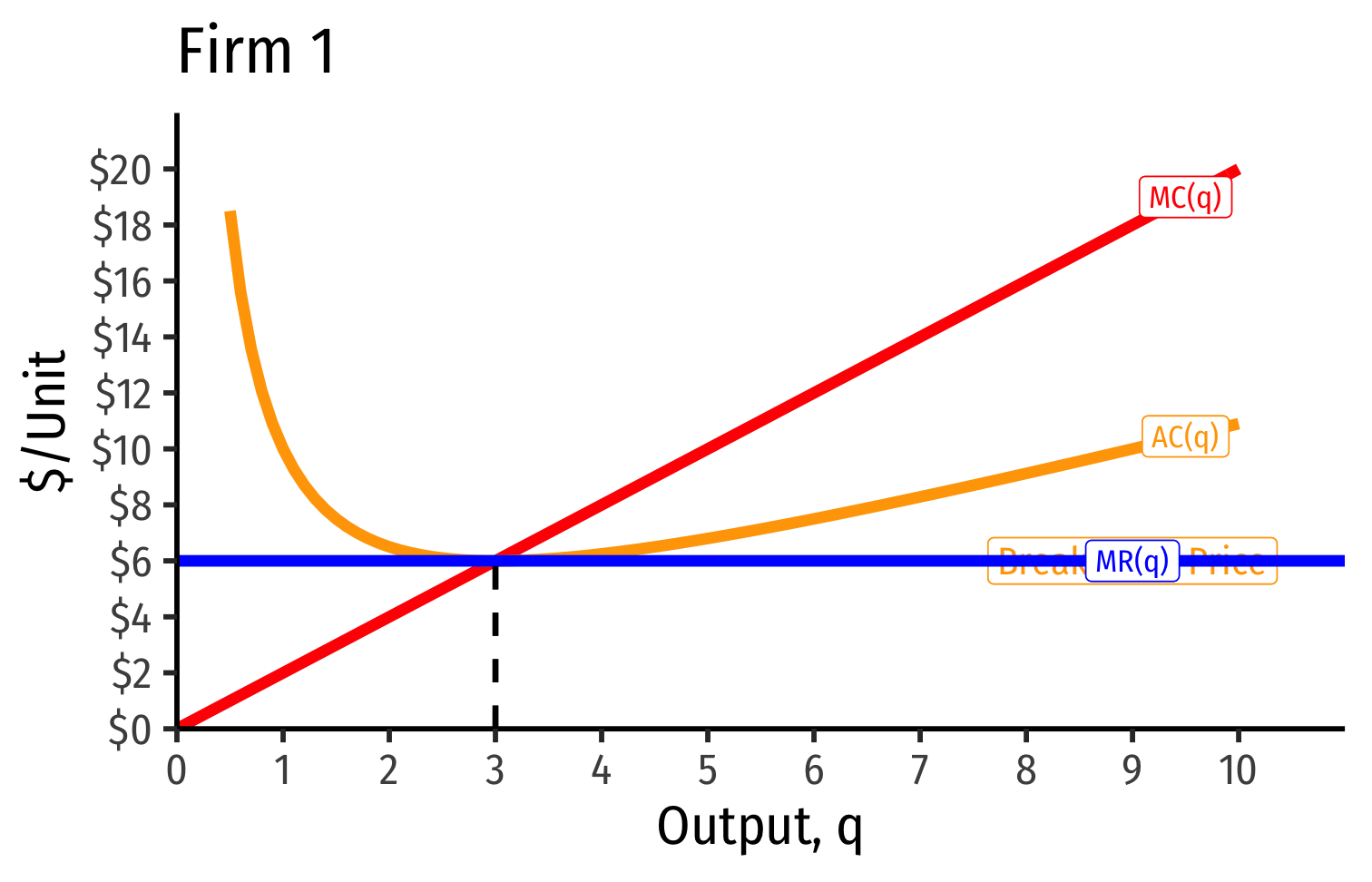
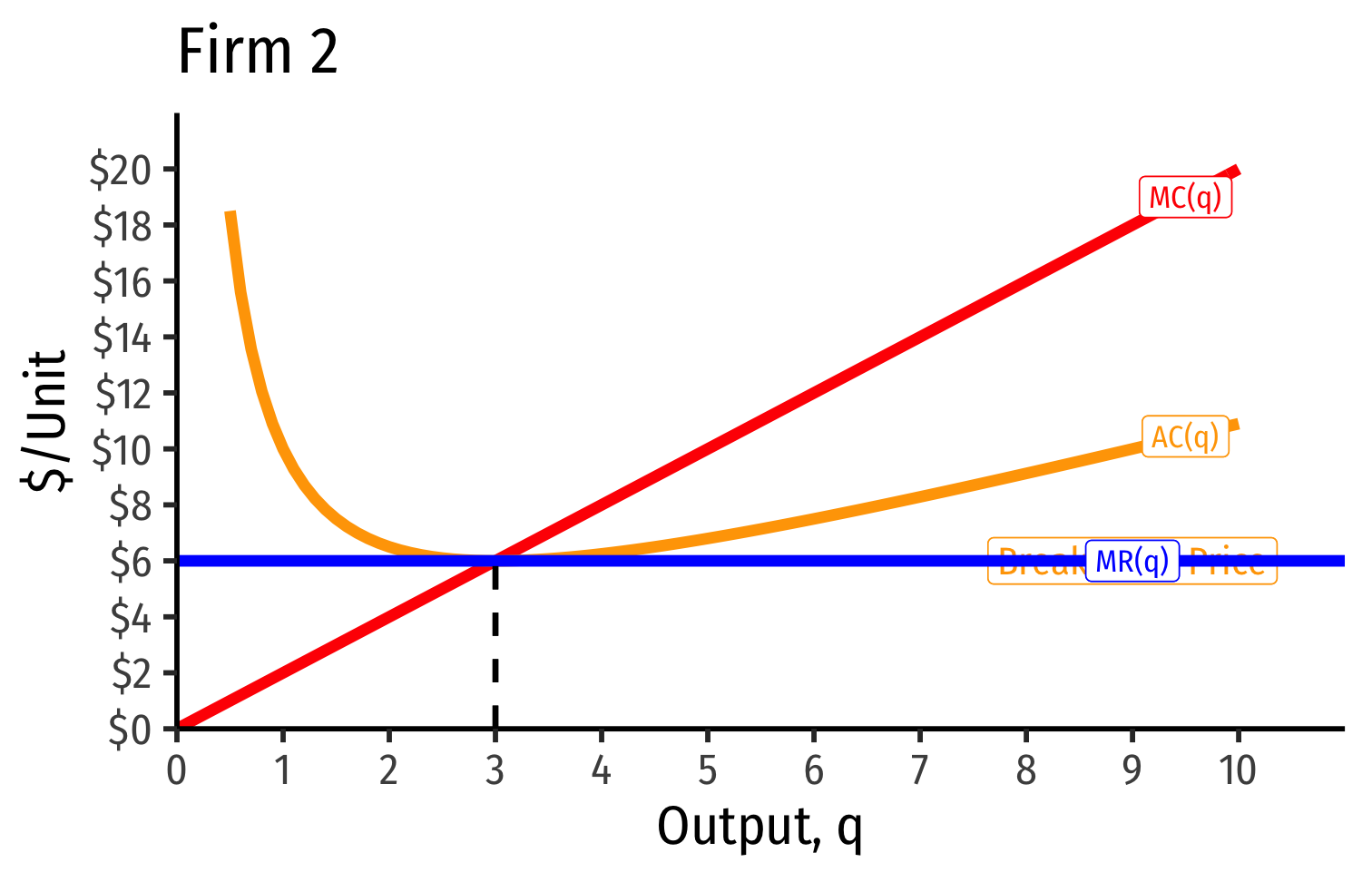
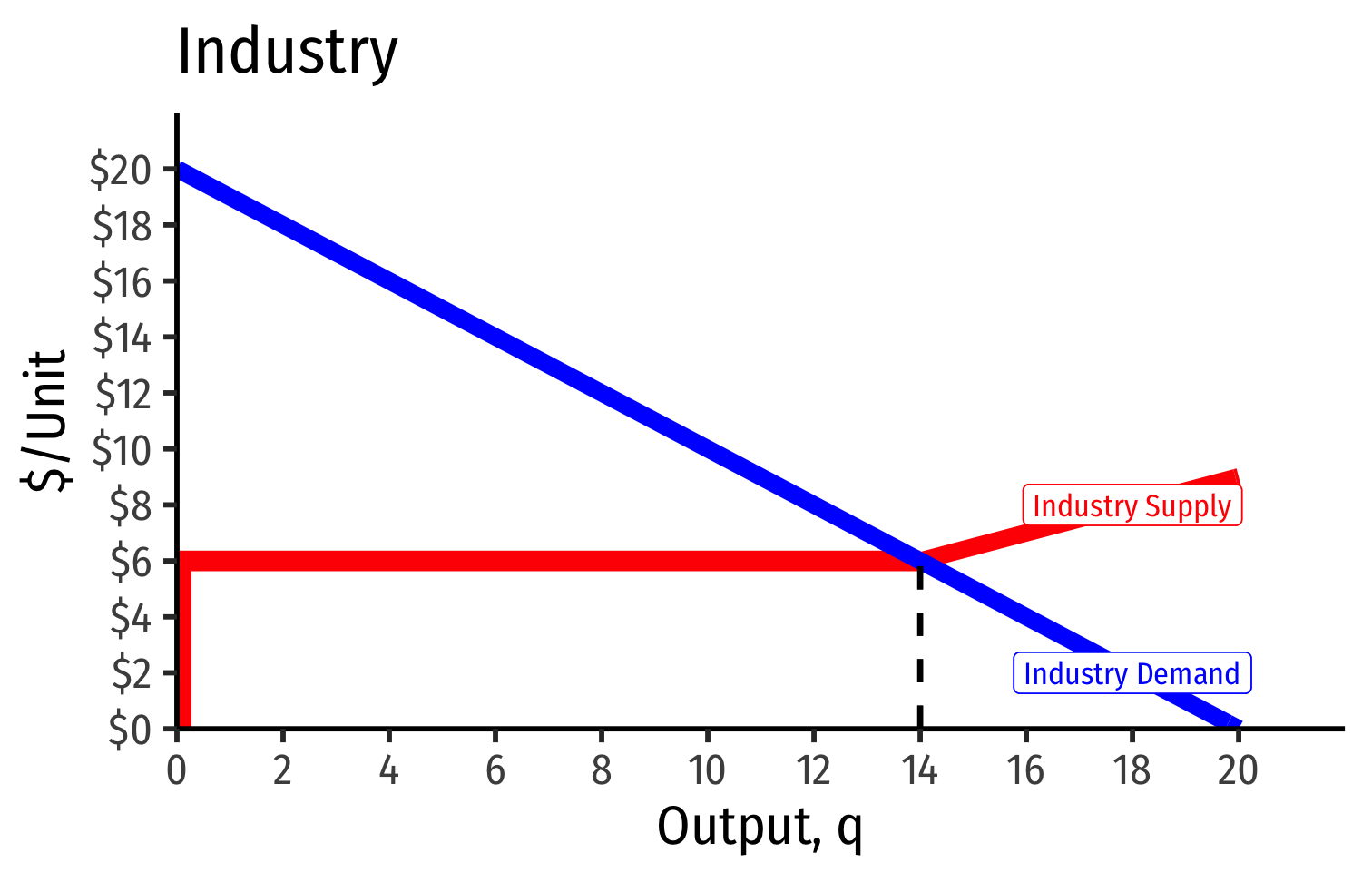
  • Short Run: each firm is earning profits p>AC(q)

  • Long run: induces entry by firm 3, firm 4, ⋯, firm n

  • Long run industry equilibrium: p=AC(q)min, π=0 at p= $6; supply becomes more elastic

Zero Economic Profits & Economic Rents

Back to Zero Economic Profits

  • Recall, we've essentially defined a firm as a completely replicable recipe (production function) of resources

q=f(L,K)

  • "Any idiot" can enter market, buy required factors, and produce q∗ at market price p and earn the market rate of π

Back to Zero Economic Profits

  • Zero long run economic profit ≠ industry disappears, just stops growing

  • Less attractive to entrepreneurs & start ups to enter than other, more profitable industries

  • These are mature industries (again, often commodities), the backbone of the economy, just not sexy!

Back to Zero Economic Profits

  • All factors being paid their market price

    • i.e. their opportunity cost - the same that they could earn elsewhere in economy

  • Firms earning normal market rate of return

    • No excess rewards (economic profits) to attract new resources into the industry, nor losses to bleed resources out of industry

Back to Zero Economic Profits

  • But we've so far been imagining a market where every firm is identical, just a recipe "any idiot" can copy

  • What about if firms have different technologies or costs?

Industry Supply Curves (Different Firms) I

  • Firms have different technologies/costs due to relative differences in:

    • Managerial talent
    • Worker talent
    • Location
    • First-mover advantage
    • Technological secrets/IP
    • License/permit access
    • Political connections
    • Lobbying

  • Let's derive industry supply curve again, and see if this may affact profits

Industry Supply Curves (Different Firms) II









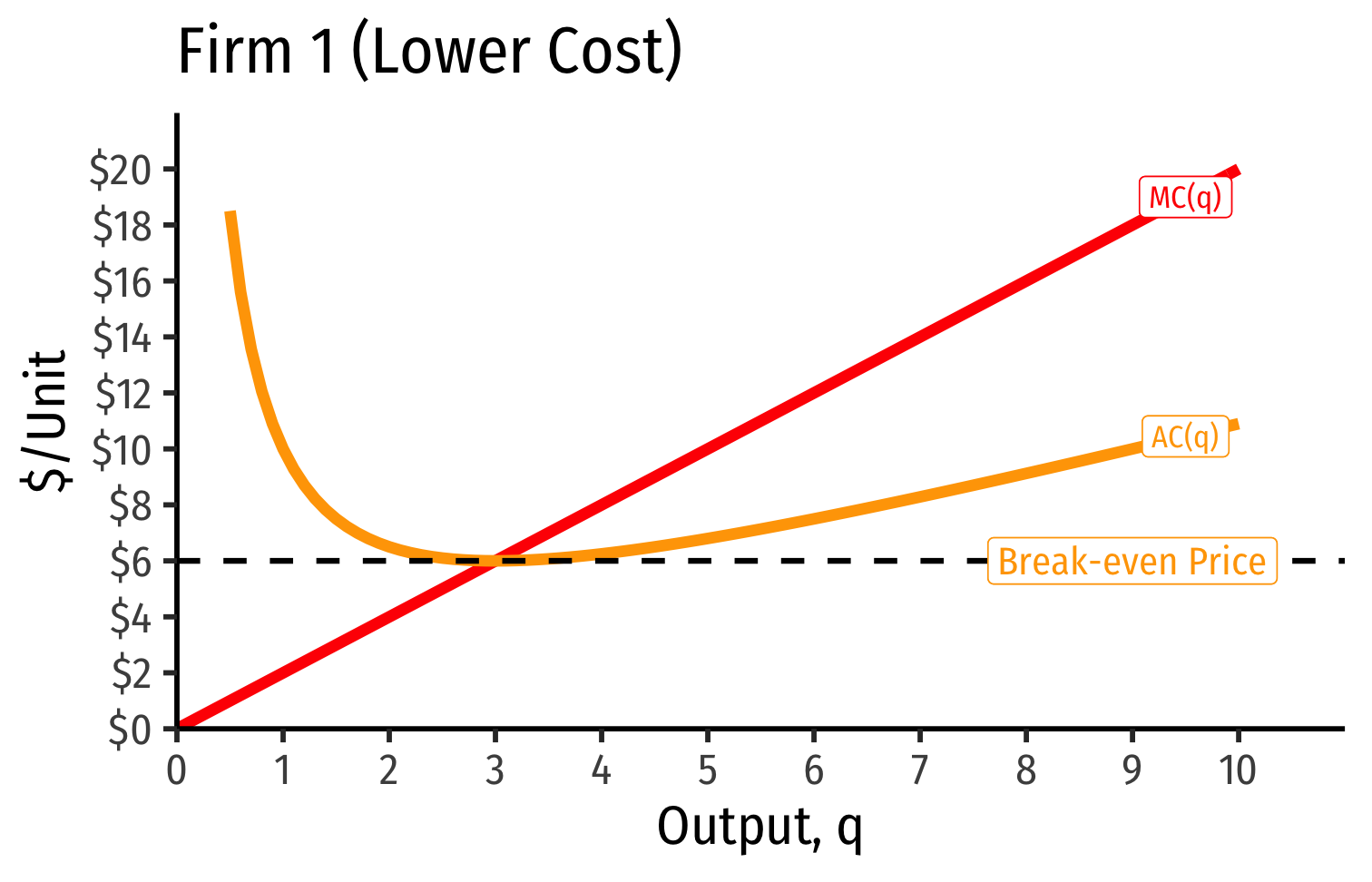
Industry Supply Curves (Different Firms) II









  • Industry supply curve is the horizontal sum of all individual firm's supply curves
    • Which are each firm's marginal cost curve above its breakeven price

Industry Supply Curves (Different Firms) II









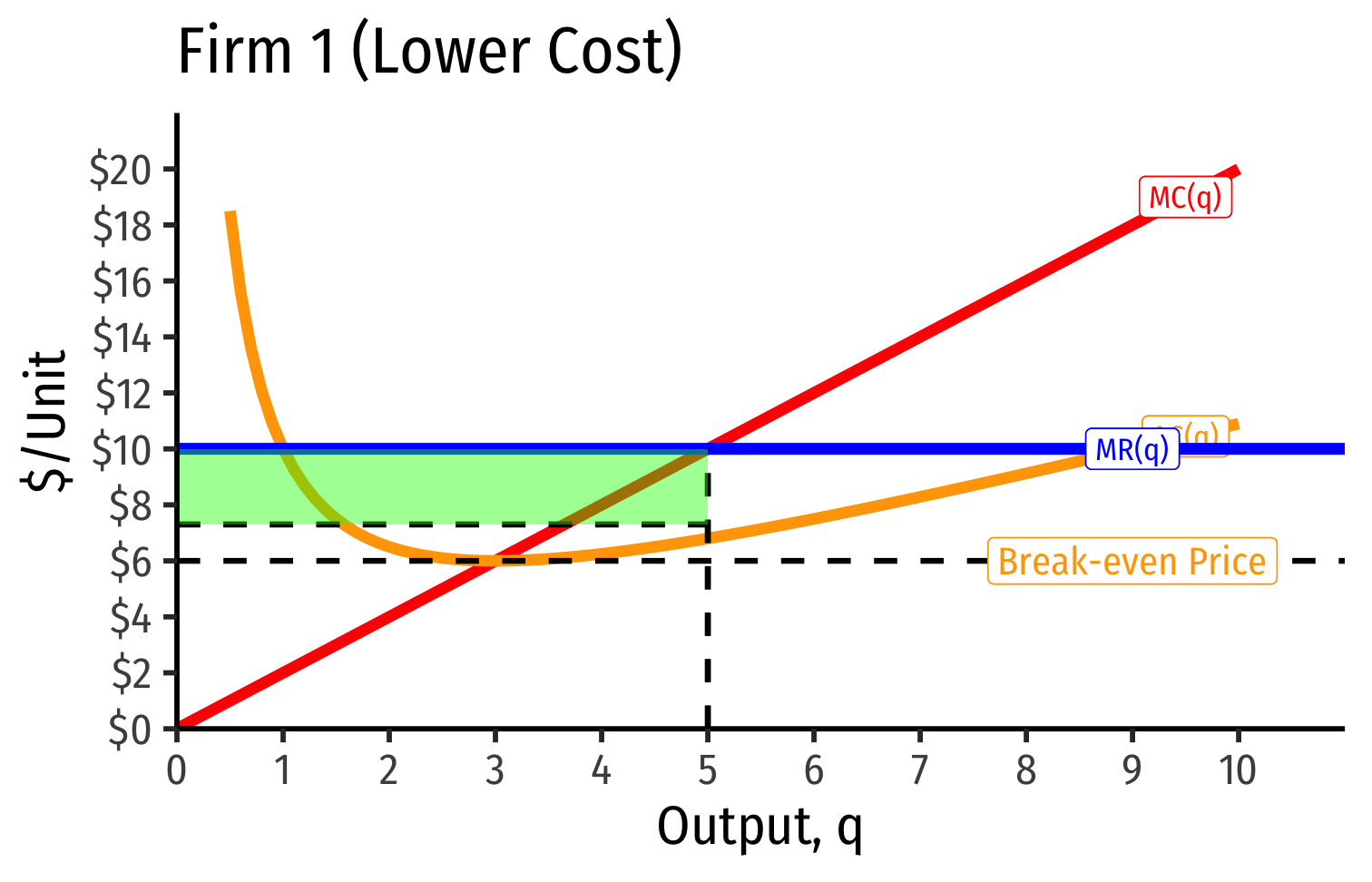
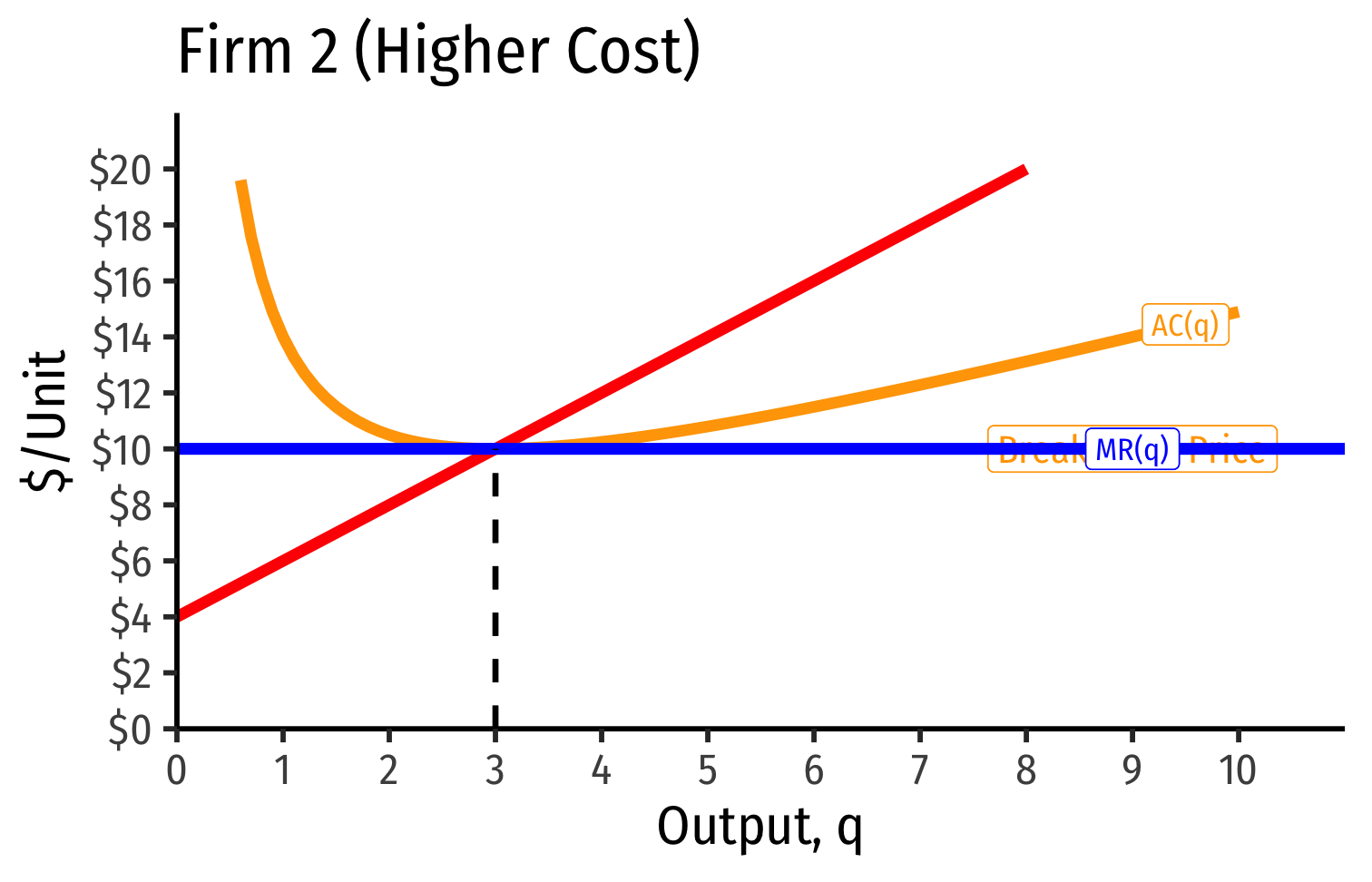
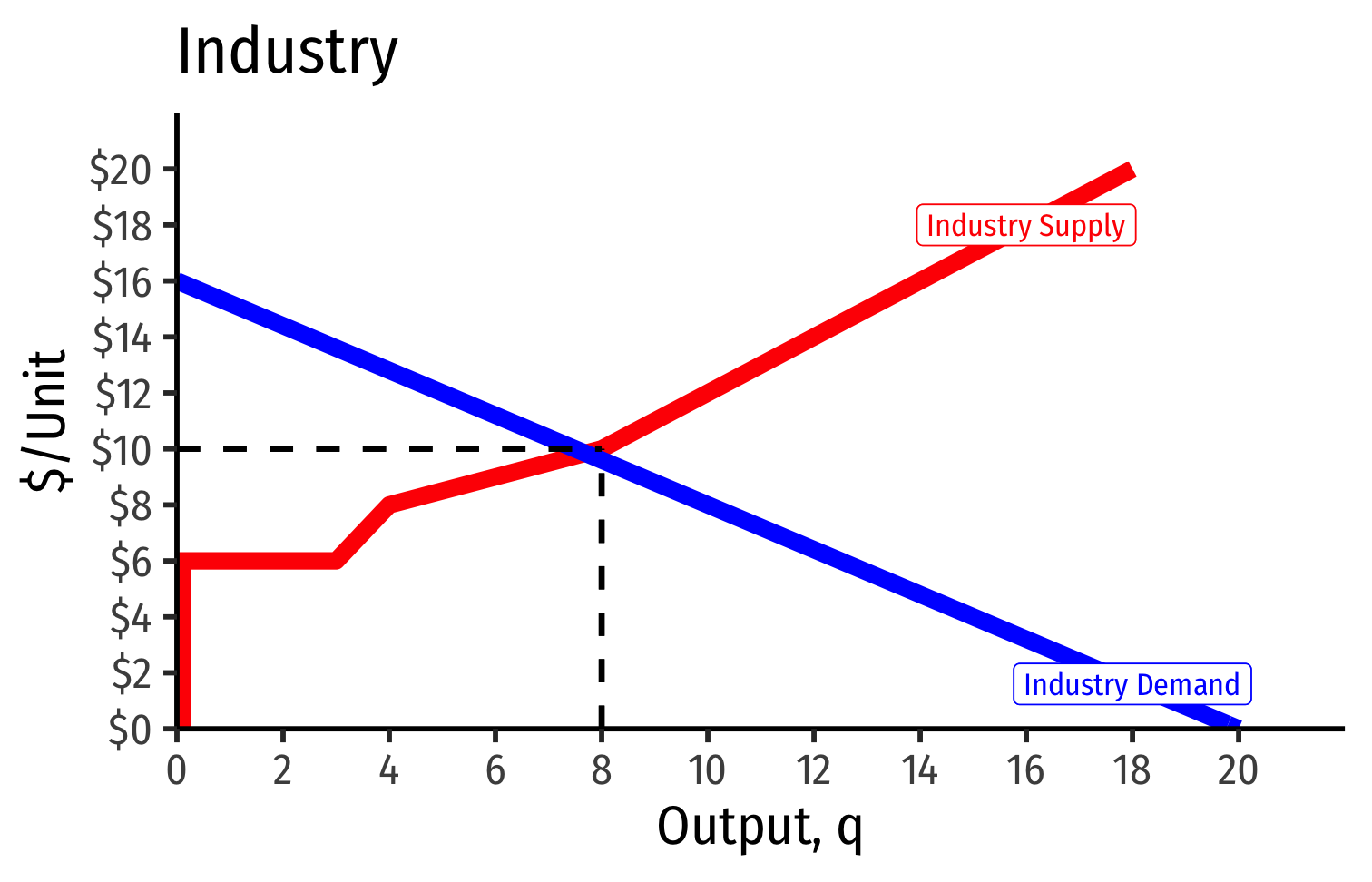
Industry Supply Curves (Different Firms) II









  • Industry demand curve (where equal to supply) sets market price, demand for firms

Industry Supply Curves (Different Firms) II









  • Industry demand curve (where equal to supply) sets market price, demand for firms
  • Long run industry equilibrium: p=AC(q)min, π=0 for marginal (highest cost) firm (Firm 2)

Industry Supply Curves (Different Firms) II









  • Industry demand curve (where equal to supply) sets market price, demand for firms
  • Long run industry equilibrium: p=AC(q)min, π=0 for marginal (highest cost) firm (Firm 2)
  • Firm 1 (lower cost) appears to be earning profits...

Economic Rents and Zero Economic Profits I

  • With differences between firms, long-run equilibrium p=AC(q)min of the marginal (highest-cost) firm
    • If p>AC(q) for that firm, would induce more entry into industry!

Economic Rents and Zero Economic Profits I

  • "Inframarginal" (lower-cost) firms earn economic rents

    • returns higher than their opportunity cost (what is needed to bring them into this industry)

  • Economic rents arise from relative differences between firms

    • actually using different inputs!

Economic Rents and Zero Economic Profits III

  • Some factors are relatively scarce in the economy

    • (talent, location, secrets, IP, licenses, being first, political favoritism)

  • Inframarginal firms that use these scarce factors gain a cost-advantage

  • It would seem these firms earn profits as other firms have higher costs...

    • ...But what will happen to the prices for the scarce factors?

Economic Rents and Zero Economic Profits IV

  • Rival firms willing to pay for rent-generating factor to gain advantage

  • Competition over acquiring the scarce factors push up their prices (i.e. costs to firms)

  • Rents are included in the opportunity cost (price) for inputs

    • Must pay a factor enough to keep it out of other uses

Economic Rents and Zero Economic Profits IV

  • Economic rents ≠ economic profits!

    • Rents actually reduce profits!

  • Firm does not earn the rents, they raise firm's costs and squeeze out profits!

  • Scarce factor owners (workers, landowners, inventors, etc) earn the rents as higher income for their scarce services (wages, rents, interest, royalties, etc).

Recall: Accounting vs. Economic Point of View

  • Recall "economic" point of view:

  • Producing your product pulls scarce resources out of other productive uses in the economy

  • Profits attract resources to be pulled out of other uses

  • Losses repel resources to be pulled away to other uses

  • Zero profits ⟹ resources should stay where they are

    • Optimal use of resources!

Supply Functions

Supply Function

  • Supply function relates quantity to price

Example: q=2p−4

  • Not graphable (wrong axes)!

Inverse Supply Function

  • Inverse supply function relates price to quantity
    • Take supply function, solve for p

Example: p=2+0.5q

  • Graphable (price on vertical axis)!

Inverse Supply Function

  • Inverse supply function relates price to quantity
    • Take supply function, solve for p

Example: p=2+0.5q

  • Graphable (price on vertical axis)!

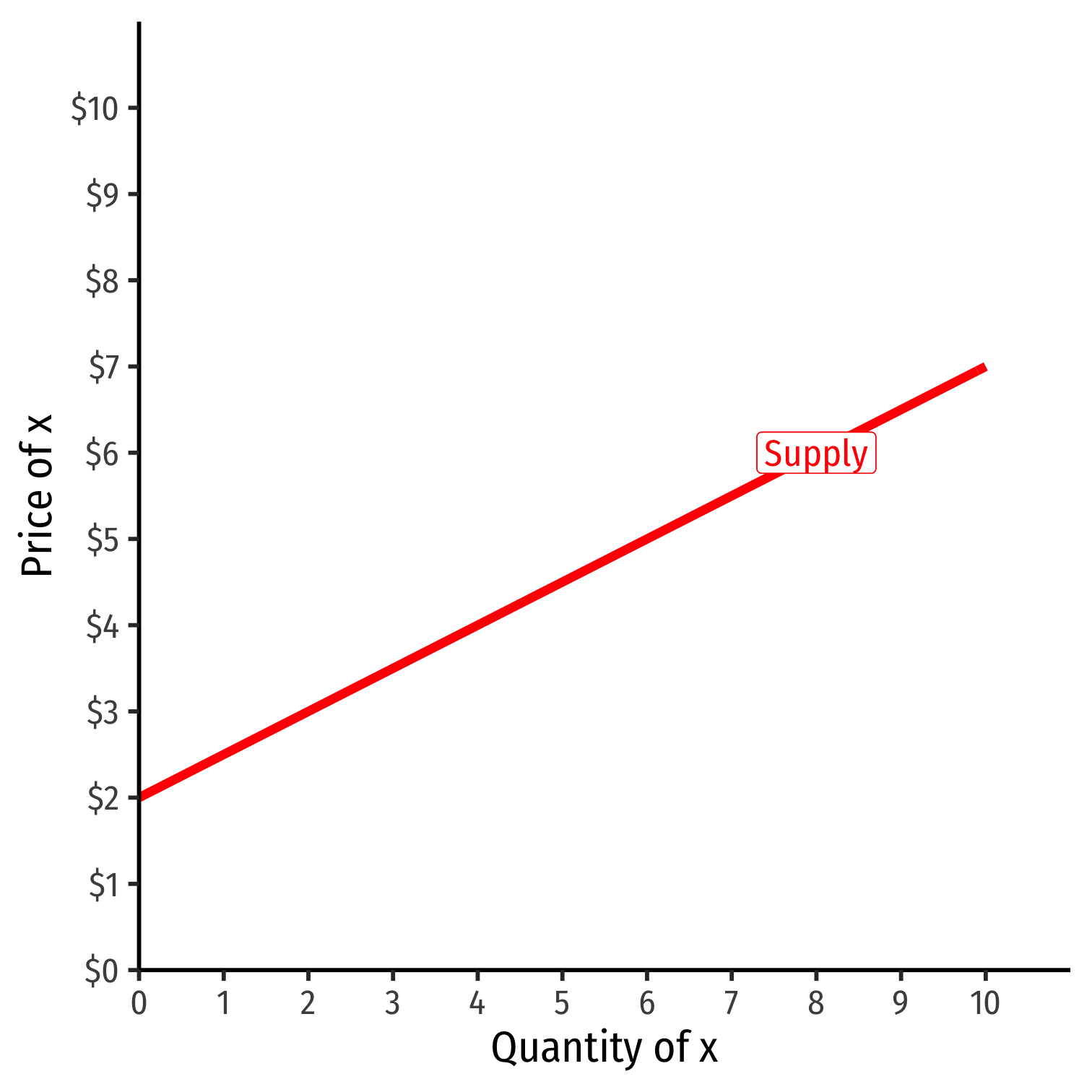
Inverse Supply Function

Example: p=2+0.5q

  • Slope: 0.5

  • Vertical intercept called the "Choke price": price where qS=0 ($2), just low enough to discourage any sales

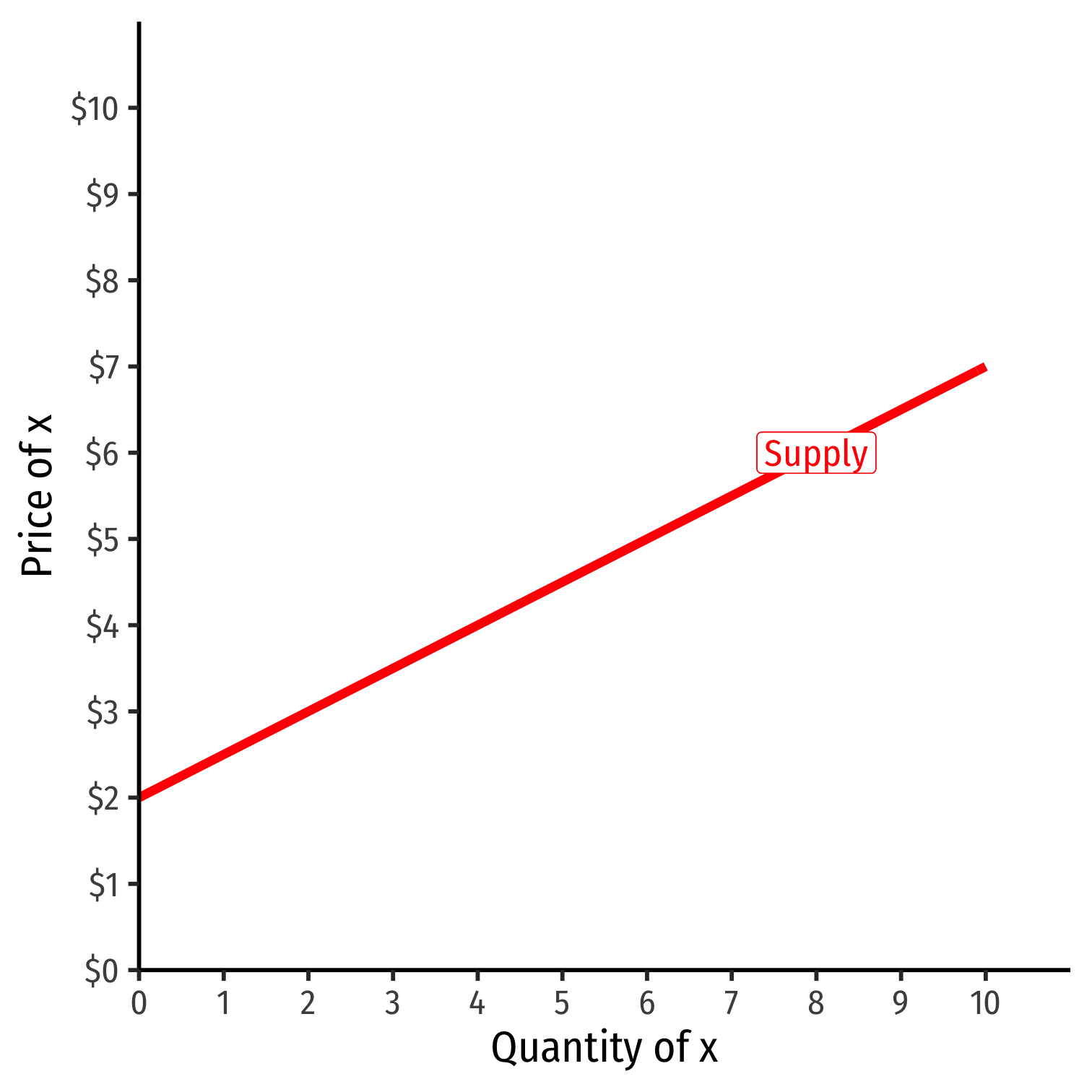
Inverse Supply Function

  • Read two ways:

  • Horizontally: at any given price, how many units firm wants to sell

  • Vertically: at any given quantity, the minimum willingness to accept (WTA) for that quantity

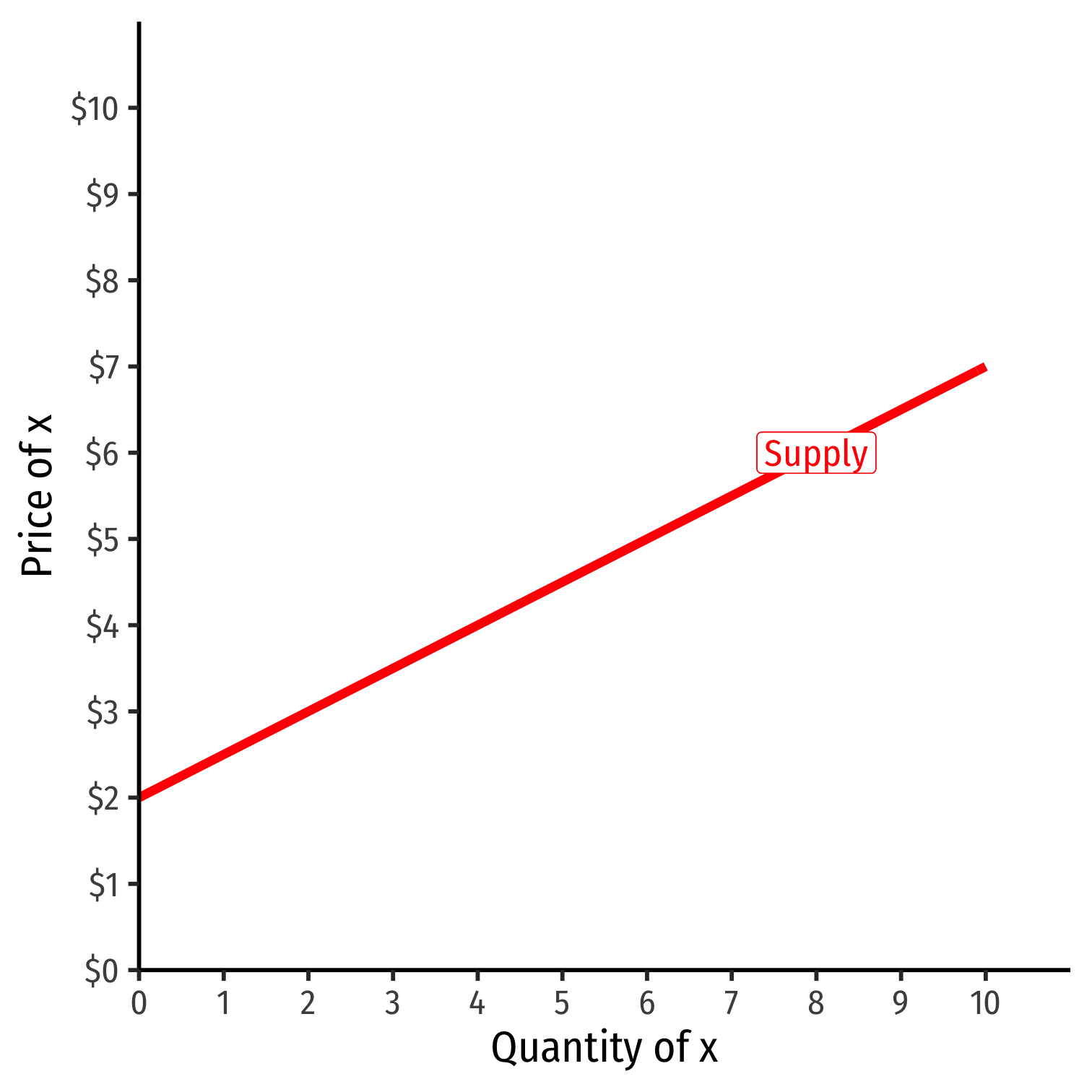
Price Elasiticity of Supply

Price Elasticity of Supply

  • Price elasticity of supply measures how much (in %) quantity supplied changes in response to a (1%) change in price

ϵqS,p=%ΔqS%Δp

Price Elasticity of Supply: Elastic vs. Inelastic

ϵqS,p=%ΔqS%Δp

"Elastic" "Unit Elastic" "Inelastic"
Intuitively: Large response Proportionate response Little response
Mathematically: ϵqs,p>1 ϵqs,p=1 ϵqs,p<1
Numerator > Denominator Numerator = Denominator Numerator < Denominator
A 1% change in p More than 1% change in qS 1% change in qS Less than 1% change in qS

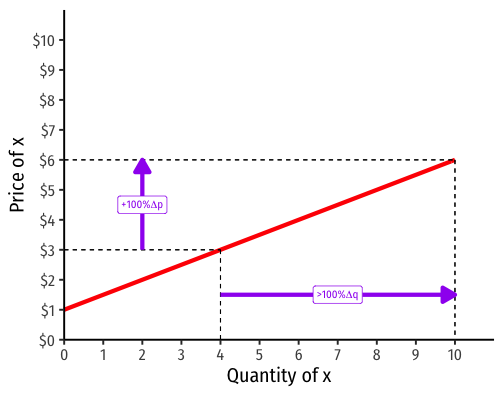
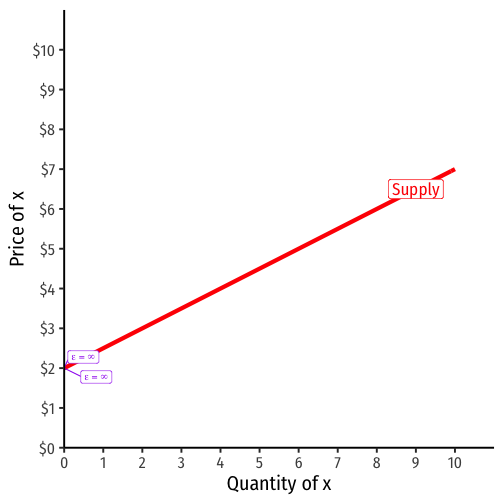
Visualizing Price Elasticity of Supply

An identical 100% price increase on an:

"Inelastic" Supply Curve

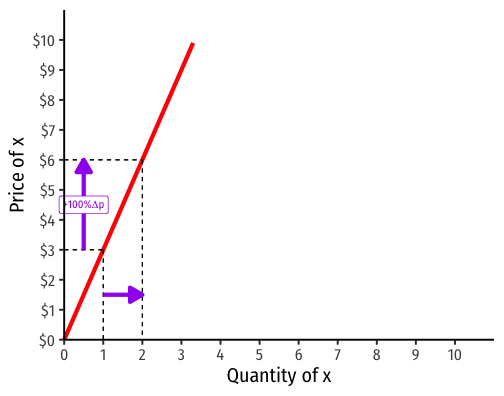
"Elastic" Supply Curve

Price Elasticity of Supply Formula

ϵq,p=1slope×pq

  • First term is the inverse of the slope of the inverse supply curve (that we graph)!

  • To find the elasticity at any point, we need 3 things:

    1. The price
    2. The associated quantity supplied
    3. The slope of the (inverse) supply curve

Example

Example: The supply of bicycle rentals in a small town is given by:

qS=10p−200

  1. Find the inverse supply function.

  2. What is the price elasticity of supply at a price of $25.00?

  3. What is the price elasticity of supply at a price of $50.00?

Price Elasticity of Supply Changes Along the Supply Curve

ϵq,p=1slope×pq

  • Elasticity ≠ slope (but they are related)!

  • Elasticity changes along the supply curve

  • Often gets less elastic as ↑ price (↑ quantity)

    • Harder to supply more

Determinants of Price Elasticity of Supply I

What determines how responsive your selling behavior is to a price change?

  • The faster (slower) costs increase with output ⟹ less (more) elastic supply

    • Mining for natural resources vs. automated manufacturing

  • Smaller (larger) share of market for inputs ⟹ more (less) elastic

    • Will your suppliers raise the price much if you buy more?
    • How much competition is there in your input markets?

Determinants of Price Elasticity of Supply II

What determines how responsive your selling behavior is to a price change?

  • More (less) time to adjust to price changes ⟹ more (less) elastic
    • Supply of oil today vs. oil in 10 years

Firm's Long Run Supply Decisions

Paused

Help

Keyboard shortcuts

↑, ←, Pg Up, k Go to previous slide
↓, →, Pg Dn, Space, j Go to next slide
Home Go to first slide
End Go to last slide
Number + Return Go to specific slide
b / m / f Toggle blackout / mirrored / fullscreen mode
c Clone slideshow
p Toggle presenter mode
t Restart the presentation timer
?, h Toggle this help
Esc Back to slideshow